His story is spellbinding. There's a really important message for you in the last two minutes ... but you won't really understand it unless you watch the whole thing.
Click on the picture. (18 min. 48 sec.)
December 29, 2006
Ben Saunders
Arctic explorer Ben Saunders recounts his harrowing solo journey to the North Pole, complete with gorgeous images, amusing anecdotes and previously unseen video footage from the Pole. At 26, Saunders became the youngest person ever to ski solo to the North Pole, updating his blog daily during the trek. He's now planning the next journey, SOUTH, an unprecedented, roundtrip expedition across Antarctica and back.
His story is spellbinding. There's a really important message for you in the last two minutes ... but you won't really understand it unless you watch the whole thing.
Click on the picture. (18 min. 48 sec.)
His story is spellbinding. There's a really important message for you in the last two minutes ... but you won't really understand it unless you watch the whole thing.
Click on the picture. (18 min. 48 sec.)
December 28, 2006
Bono - TED Prize Winner
For the past 20 years, members of the TED community have gathered together to share ideas and passions that are big enough to change the world. Each year they will honor a maximum of three individuals who have shown that they can, in some way, positively impact life on this planet.
Rather than simply receiving financial support, winners of the TED Prize are granted something extraordinary: something which children dream about, but which adults assume is merely the stuff of fairy-tales.
They are granted three WISHES to change the world.
They may wish for anything. And TED will seek to make their wishes come true.
Bono won the TED prize in 2005.
Rock star and activist Bono accepts the 2005 TEDPrize with a riveting talk about our moral obligation (and economic incentive) to help lift Africa out of poverty. He unveils his TEDPrize wishes by challenging the TED community to help build a social movement of more than one million American activists for Africa; to tell people one billion times about the ONE campaign; and to connect every hospital, health clinic, and school in one African country, Ethiopia, to the Internet.
Click on the picture. (28 min. 37 sec.)
For more: Read the update on Bono's wishes.
Rather than simply receiving financial support, winners of the TED Prize are granted something extraordinary: something which children dream about, but which adults assume is merely the stuff of fairy-tales.
They are granted three WISHES to change the world.
They may wish for anything. And TED will seek to make their wishes come true.
Bono won the TED prize in 2005.
Rock star and activist Bono accepts the 2005 TEDPrize with a riveting talk about our moral obligation (and economic incentive) to help lift Africa out of poverty. He unveils his TEDPrize wishes by challenging the TED community to help build a social movement of more than one million American activists for Africa; to tell people one billion times about the ONE campaign; and to connect every hospital, health clinic, and school in one African country, Ethiopia, to the Internet.
Click on the picture. (28 min. 37 sec.)
For more: Read the update on Bono's wishes.
December 27, 2006
Richard St. John
Why do people succeed? Richard St. John compacts seven years of research into an unmissable 3-minute slideshow on the real secrets of success (Hint: Passion, persistence, and pushy mothers help).
Click on the picture. (3 min. 40 sec.)
Click on the picture. (3 min. 40 sec.)
December 26, 2006
Gregory Colbert
Photographer Gregory Colbert shares the remarkable images and film footage from his exhibit, "Ashes and Snow," and announces his founding of the Animal Copyright Foundation, which will require that royalties be paid when images of nature are used for commercial purposes.
The pictures are just stunning.
Click on the picture. (18 min. 42 sec.)
The pictures are just stunning.
Click on the picture. (18 min. 42 sec.)
December 25, 2006
Hans Rowling
Hans Rowling is a professor of of international health at Sweden's world-renowned Karolinska Institute.
Watch how he displays and talks about statistics. He'll make you laugh and he'll make you think.
Click on the picture. (20 min. 35 sec.)
December 22, 2006
Scribe news
Hi it's me benofschool and m@rk will be finishing up his scribe post over the holidays and he has told me that I will be the next scribe. So therefore I will be the first scribe in 2007 for our pc30sf06 blog. Until 2007 Merry Christmas and happy holidaze!!
Don't Let This be You ...
Have an excellent holiday ... but try to do some math too ... you don't want this to be you do you?
Cheers,
Mr. K.
Cheers,
Mr. K.
Flickr Assignment Rubric v1.0 - We're Out of Beta!
Here is the rubric we've settled on together.
Thanks to everyone who helped put this together. I found this to be a great experience for me as a teacher I hope it was also valuable to you as a student.
Anyway, here it is, version 1.0 fresh out of beta. ;-)
Flickr Assignment Rubric
It is paramount that the picture be in tune with the purpose of the assignment. It should show, first of all, the student's understanding of how the photo is related to mathematics. The hot spots are important too, because that's essentially your way of teaching other people. Creativity is a factor, because keeping one's interest in the photo contributes to the learning process. Finally, the picture quality should be kept in mind too. If we can't see the picture, it's going to be hard achieving all the other requirements.
Tags
The picture must be tagged properly with the course tag and assignment tag. If tags are misspelled or no tags are present the photo cannot be graded and will receive a grade of ZERO. Not tagging your photo properly and accurately is analogous to not handing in your work or not putting your name on it.
| Classification | Mathematical Content (50%) | Hot Spots (35%) | Photograph (15%) |
| Level 4 | Packed with mathematical concepts/facts. (Minimum 7 concepts/facts.) | All hot spots accessible; i.e. "smaller" hot spots are "on top" of larger ones, they do not obscure each other. All hot spots are actually labels and relate to parts of the photo (not on blank space with filled in notes). One or more hot spots include a link to a relevant supporting resource on the internet. Minimum 7 hot spots. | In focus or appropriately focused for effect. The subject of the picture occurs "naturally," it is not a contrived shot. Really makes the viewer "see" math in a place they hadn't realized it existed. (Example: trigonometry) |
| Level 3 | Significant number of concepts/facts included. (Minimum 5 concepts/facts.) | All hot spots accessible. Most hot spots are actually labels and relate to parts of the photo. Not more than one hot spot on blank space. One or more hot spots may include a link to a relevant supporting resource on the internet. Minimum 5 hot spots. | In focus or appropriately focused for effect. The subject of the photo has been "set up" or contrived yet still illustrates math found in "the real world." (Example: derivative) |
| Level 2 | Some effort to include content evident. (Minimum 3 concepts/facts.) | Most hot spots accessible. Most hot spots are actually labels and relate to parts of the photo. More than one hot spot is on "blank" space. May or may not include links to relevant supporting resource on the internet. Minimum 3 hot spots. | In focus or appropriately focused for effect. Although it is a "real world" picture, objects have been used to "draw" the math. An obviously contrived shot. (Example: trigonometry) |
| Level 1 | Very scarce content related to assignment. | Less than three hot spots are visible or have information related to the theme of the assignment. | It is evident that little effort went into finding and shooting a picture that reflects the theme of the assignment. |
| Level 0 | Content unrelated to theme of assignment. | No hot spots or mostly unrelated to the theme of the assignment. | Out of focus and/or otherwise difficult to look at. |
Creativity (up to 5% bonus)
The maximum possible mark for this assignment is 105%. You can earn up to 5% bonus marks for being creative in the way you approach this assignment. This is not a rigidly defined category and is open to interpretation. You can earn this bonus if your work can be described in one or more of these ways:
- unique and creative way of looking at the world, not something you'd usually think of;
- original and expressive;
- imaginative;
- fresh and unusual;
- a truly original approach.
December 21, 2006
First things first? One day left to go--
Wondering if you're having trouble putting first things first in these last days before winter break?
And
Wanting to wish each of you happiness, peace, and joy during this season! Be safe and enjoy!
Best,
Lani
December 20, 2006
BOB v.5 BcircleB Circle Geometry
When I first thought about Circle Geometry, I first didn't know what the unit will be about, but as we continued on with the unit, I found out it was about the angles and lines which make up theorems. Doing the investigations were easy and straightforward because all we had to do was follow the instructions...but in the end, we had to memorize them all! Yeah, so circle geometry was really straightforward. The only difficult thing about this unit is where you have to prove something which can take a LOT of time to do and that's the only part I'm worried about. My goal is to get at least a 70% or above on the test (depending on how many "proof" questions there are) which is coming up in about...less than two hours!! XD So I'll cya all in 6th period!
December 19, 2006
" L O G i C " - cont'd
To start off today's class, we were given a few reminders:
- FLICKR.com RUBRIC: Reminder to students that this is for your benefit. Putting in your voice nto this document will affect how your flickr assignments are graded. This document should be about how you want your assignments to be marked, and how the assignments should be done. Get crackin'!
- NEXT FLICKR ASSIGNMENT: The next flickr assignment will be to take a picture of TRIGONOMETRY. Be unique and creative and find a photo no one else will have. This won't be due until the first tuesday after winter break. We'll be given a great amont of time for this assignment, but don't procrastinate!
Today, we added in some more notes into our math dictionary:
Here are a few examples of some arguments:
Example 1 and 2 both are true and valid statements. The reason being the premises of each example makes sense with what the conclusion says. The conclusion makes sense of what has been stated and flows naturally. This is called a sound argument.
SOUND ARGUMENT: an argument that is both valid and true.
AN EXAMPLE OF A FALSE ARGUMENT:
"All men are mortal.
Mr. K is a man
... Mr. K wears glasses."
That was a false statement because, even if the three statements alone are true, together as an argument, it's not. The conclusion doesn't flow naturally with the premises, and it doesn't make sense.
**************************************
We also discussed different TYPES OF REASONING that we go over in this unit of Logic:
Induction: When we observe several particular examples that identify a pattern and conjecture that it must always be that case.
EXAMPLES:
QUESTION: Will the sun rise tomorrow?
ANSWER: It has every day before, so it will rise again tomorrow.
*That's the answer because through all the years we've been living, a reoccurring pattern of the sunrise has always taken place.
NOTE: To view more examples of inductive reasoning, look over all the investigations we worked on in our circle geometry unit!
Deduction: When we argue from basic, unarguable truths, to a valid conclusion.
An example of deductive reasoning is the process of proving THALES' THEOREM.
***************************************
In logic, we also look at relationships between different sets, and compare them in Venn Diagrams.
In the following images, we see how sets are made, and how we can compare two different sets using a venn diagram.
***************************************
Weeell ..
TOMORROW'S THE DAY! CIRCLE GEOMETRY UNIT TEST
I HOPE YOU GUYS ENJOYED MY SCRIBE..
blogger was being a pain in the behind and erased my
first draft and I had to start all over again!
BUT, I DID IT AGAIN. YET ANOTHER SCRIBE POST BY ME!
TOMORROW'S SCRIBE WILL BE ...
M@RK.
(just cuz he told me to pick him!)
NiGHT!!
Circle Geometry Review
We're gearing up for our test tomorrow. Here's a bunch of online quizzes you can use to help get ready. Have you added any really good links to our del.icio.us box lately? ;-)
You can find circles in the most unusual places ...
- Proof of Congruence (5 questions - refresh the page for more quizzes)
- More Congruence (5 questions - refresh the page for more quizzes)
- Circles (5 questions - refresh the page for more quizzes)
- Arcs &Angles (5 questions - refresh the page for more quizzes)
- Arcs & Chords (5 questions - refresh the page for more quizzes)
- Inscribed Angles (5 questions - refresh the page for more quizzes)
- Tangents (5 questions - refresh the page for more quizzes)
- Polygons (5 questions - refresh the page for more quizzes)
Whew! More quizzes than you can shake a stick at! ;-)
December 18, 2006
New Unit: Logic
So today we started logic which is a system of reasoning or the way you present an argument.
Mr.K asked us if the sun is going to rise tomorrow and if i'm not mistaken almost everyone thinks that the sun is going to rise tomorrow based on the fact that the sun has been setting and rising every single day of our lives so far. That brings us to inductive reasoning and deductive reasoning
Inductive reasoning is when you come up with a hypothesis based on examples or observations to make a general rule more specific.
Deductive reasoning is when you use fundamental trues to create a rule. An example of deductive reasoning is Thales' theorem.
Something like this would be inductive:
Draw the next two.
With the next two it would look like this:
Something like this would be deductive:
Draw the next figures.
There really is no right answer for this question, you can look for a pattern but you must explain 3 things: the square, the curve, and the dot.
Here is a true or false questions:
1. The square of a number is larger than the number.
- this is false because the square of 1 is not larger than 1 (12 = 1)
A sentence like "This sentence is false." is what we call a paradox. It is true and false because it refers to two things at the same time and it is a self-reference.
valid means it flows natuarally from the premisis.
invalid means it does not flow naturally from the premisis.
All men are mortal
Mr.K is a man
Mr.K is mortal
^^ This is what we call a sound argument because it is both true and valid
A few examples of unsound arguments are:
All men are squibbles
Squibbles are pink
All men are pink
^^ This is unsound because it is valid but it is false.
All men are mortal
Mr.K is a man
Mr.K wears glasses
^^ This is unsound because it is all true but it is also invalid
All men are green
Mr.K is a man
Mr.K is green
^^ Something like this is also unsound because it is valid but most of it is false
SO THAT IS WHAT WE DID TODAY. HOMEWORK IS EXERCISE 45
&&
CONSUMER MATH - WAGES ASSIGNMENT **due on friday
NEXT SCRIBE IS LAURESSA
Mr.K asked us if the sun is going to rise tomorrow and if i'm not mistaken almost everyone thinks that the sun is going to rise tomorrow based on the fact that the sun has been setting and rising every single day of our lives so far. That brings us to inductive reasoning and deductive reasoning
Inductive reasoning is when you come up with a hypothesis based on examples or observations to make a general rule more specific.
Deductive reasoning is when you use fundamental trues to create a rule. An example of deductive reasoning is Thales' theorem.
Something like this would be inductive:
Draw the next two.
With the next two it would look like this:
Something like this would be deductive:
Draw the next figures.
There really is no right answer for this question, you can look for a pattern but you must explain 3 things: the square, the curve, and the dot.
Here is a true or false questions:
1. The square of a number is larger than the number.
- this is false because the square of 1 is not larger than 1 (12 = 1)
A sentence like "This sentence is false." is what we call a paradox. It is true and false because it refers to two things at the same time and it is a self-reference.
valid means it flows natuarally from the premisis.
invalid means it does not flow naturally from the premisis.
All men are mortal
Mr.K is a man
Mr.K is mortal
^^ This is what we call a sound argument because it is both true and valid
A few examples of unsound arguments are:
All men are squibbles
Squibbles are pink
All men are pink
^^ This is unsound because it is valid but it is false.
All men are mortal
Mr.K is a man
Mr.K wears glasses
^^ This is unsound because it is all true but it is also invalid
All men are green
Mr.K is a man
Mr.K is green
^^ Something like this is also unsound because it is valid but most of it is false
SO THAT IS WHAT WE DID TODAY. HOMEWORK IS EXERCISE 45
&&
CONSUMER MATH - WAGES ASSIGNMENT **due on friday
NEXT SCRIBE IS LAURESSA
December 17, 2006
BOB; circles
Circle Geometry was by far the hardest unit yet. It was alright at first because we went through it as a class, step by step. Although, I didn't always understand the investigation because of my poor measuring skills. But, when all ten investigations were done I finally understood it all. I find it more easier for me to understand things when I'm told of what to look for and practicing it, rather than finding it out myself. Most of the investigations, I didn't know what I found because my measuring skills aren't so good.
I'm happy that I reviewed before the pre-tests and quizzes because that's where it came in place, piece by piece I began to understand what was going on and why different angles are the same or two times larger. This happened on Thursday I believe. It was like someone had replaced a light bulb to the lighthouse in my head. However, I wasn't prepared enough. So, my options are study harder or study harder (haha).
The up side is, I understand the theorems and I look for different triangles and angles. The down-side is, sometimes I doubt myself and have second thoughts about some things, which makes me to change my answers (which were right in the beginning). But, most of the things, I understand. Well, I hope that everyone does well and studies hard. See you all tomorrow fellow class mates!
-SAMUS
I'm happy that I reviewed before the pre-tests and quizzes because that's where it came in place, piece by piece I began to understand what was going on and why different angles are the same or two times larger. This happened on Thursday I believe. It was like someone had replaced a light bulb to the lighthouse in my head. However, I wasn't prepared enough. So, my options are study harder or study harder (haha).
The up side is, I understand the theorems and I look for different triangles and angles. The down-side is, sometimes I doubt myself and have second thoughts about some things, which makes me to change my answers (which were right in the beginning). But, most of the things, I understand. Well, I hope that everyone does well and studies hard. See you all tomorrow fellow class mates!
-SAMUS
B.O.B.
Once again, a unit finished.
This semester is almost over too. (tear*)
The circle geometry unit was a lot of fun. I really liked this unit, mostly because it involves looking at a real image and trying to figure out measures. I like how everything all connects, when you find one part you can also find another. The trouble I have is remembering the theorems. I'll just have to look over my math dictionary more. That's all for now, good luck!!
This semester is almost over too. (tear*)
The circle geometry unit was a lot of fun. I really liked this unit, mostly because it involves looking at a real image and trying to figure out measures. I like how everything all connects, when you find one part you can also find another. The trouble I have is remembering the theorems. I'll just have to look over my math dictionary more. That's all for now, good luck!!
BOB
I actually found this unit to be the easiest out of all the other units we've worked on. I have the whole concept down on what Circle geometry is all about. Remembering the names of the theorems is probably the only thing I have to get used to. Other than that, I think I'll finally be able to get this test down and get a well rounded mark from it. I hope it's the same with everyone else. Study hard, and Good luck!
December 16, 2006
Circle Geometry: Theorem Summary
Okay, so I was responsible in making the first post dedicated to Circle Geometry and now I'm responsible in making the last post dedicated to Circle Geometry. Just like the first post, the following information is the "Dictionary Information."
The usual human brain can only remember at max 7 items, according to Mr. K. In remembering a phone number, we chunk the 7 digits into 2 groups so its easier to remember. Just like remembering a phone number, we chunk all the theorems into 4 categories.
next scribe is crysta! go crysta! i'm sure you'll find some new innovative way for learning just like what u did with that bubbleshare thingy! =)
The usual human brain can only remember at max 7 items, according to Mr. K. In remembering a phone number, we chunk the 7 digits into 2 groups so its easier to remember. Just like remembering a phone number, we chunk all the theorems into 4 categories.
next scribe is crysta! go crysta! i'm sure you'll find some new innovative way for learning just like what u did with that bubbleshare thingy! =)
December 15, 2006
BOB
Well, I think that this unit is pretty much straight forward for me. I think that I can do better in this test if we only practice more or at least spend one more class using the theorems.The name of the theorems doesn't really stick on my mind so I devise a naming method myself. Hopefully we can all have an excellent mark in this test.
******BREAKING NEWS******
THe test is postponed FOR MONDay.
*********************************
******BREAKING NEWS******
THe test is postponed FOR MONDay.
*********************************
December 14, 2006
BOB - Anatomy of Circles
Well well well. Another test, another BOB. I find myself saying that in every BOB entry I write. Anatomy of Circles... well it hasn't been my favourite unit but more of a reason to look foward to our test tomorrow (so it'll be the end of it). I don't know why but I guess this whole theory of circles is something I find hard to understand and process in my brain by myself. We'd be in class and Mr. K goes over it step by step and it seems to be okay. I understand the rules and different theoroms when I'm in class. However when I get home and is presented a question- I sometimes dont even know where to start. I guess it is the fact there is a broad number of ways to solve any given question. Well, hopefully we all pull through the test tomorrow. As for myself I don't expect myself to do incredibly well :(, since it is one of my weaker units.
WELL GOOD LUCK AND GOOD NIGHT :)
11 days until christmas!
WELL GOOD LUCK AND GOOD NIGHT :)
11 days until christmas!
BOB - circle geometry
Yet another unit test. I was supposed to have two tests tomorrow. One for pre cal, and one for chemistry. Luckily! my chemistry has been postponed to monday. haha. Well, this unit was really confusing for me. I found it difficult at first to prove everything. And i still haven't memorized all those theorems. :\ Well, lots of cramming tonight! Anyway, i'd like to become like, pro at this. so i'm going to keep practicing to keep myself familiar with it. Besides that everything is fine. I've spent my whole evening besides my brothers christmas concert on math, so it's time for my break. Happy holidays guys! And again, good luck tomorrow on the test. =)
Circle Geometry - Pretest
Today we did our pre test in class for Circle Geometry. More or less i think the whole class found that the long answer question and the last multiple choice question were the hardest, so i'll explain those. =)
LONG ANSWER
Determine the measure of ےECB, ےBDC, ےBAD, and ےDBE, where E is the centre of the circle.
So first of all we always start with a table that has STATEMENT on one side and REASON on the other.
It's obvious at this point that BE and CE are radii, therefor triangle BCE is an isosceles triangle. This also means that ےEBC and ے BCE are the same.
NOTE: CLICK ON BUBBLESHARE BOX TO SEE FULL EFFECTS. =)
We now know the exact amount of ےBCD and according to cyclic quadrilateral theorem ے BCD's and ےBAD's sum should equal 180°. So in order to find ےBAD, we subtract 30° and 40° from 180° and come up with 110°. Therefore ےBAD is 110°.
Another thing we looked at were the two triangles that are subtended by the same arc; triangle BCE and triangle BCD. Since all of us know of the central inscribed angle theorem ( AKA the star trek theorem ) we can easily point out that ےBDC is half the amount of ےBEC. As a result ےBDC = 100°/2 which equals 50°.
We only have one angle left! Angle DBE! So if we look closely at triangle BCD you'll realize that we only have one angle left to find, ےDBE. We also know that every the angles of a triangle sum up to be 180° and by subtracting every angle that we have we'll be able to come up with the value of ےDBE! :D
Which in this case in 20°! =)
Once you've found your information and proofs, your table (including "Statement" and "reason") should look something like this:
MULTIPLE CHOICE
In the diagram below, R, S, T, U are points on the circle with RS = RU = UT and SU = ST. Find the measure of ےUST.
a) 31°
b) 35°
c) 36°
d) 40°
e) none of these
The first thing you should always do is mark down what is given to you. Have a wishful thinking colour, a colour used for given facts and a colour used for things you have proven! ( it comes in handy :D )
I'll use purple as the facts that are given, blue for what we're looking for and orange for the facts i prove. =)
There's no particular order to do this in, but what i looked at first was the isosceles triangles. We can easily point out that ےRSU and ےRUS are the same and ےSUT = ےSTU.
Now if we look at RS, RU and UT they are all the same length. Which means that the angles subtended by this chord length will all be the same. SOOO.. ےRST = ےRUT = ےUST.
In order to find the exact degree that angle is we make three equations. (this is like going back to substitution in linear equations). Here are the three equations (let ےSRT = w):
1. w + z = 180
2. 3y + z = 180 --> z = 180 - 3y
3. w + 2y = 180 --> w = 180 - 2y
If we substitude 2 and 3 into 1, we can find out what Y is, which is ےUST.
180 - 2y + 180 - 3y = 180
180 + 180 - 180 = 2y + 3y
180 = 5y
36 = y
And there you have it!
c) 36° is the correct answer. =)
That's all for tonight! Sorry for the late entry, i had to go and watch my brother's christmas concert! haha. =) Well, no homework tonight, just study! Test tomorrow. Good luck guys!
HAPPY HOLIDAYS! =]
And the next scribe will bee..... -Zeph! Enjoy. =)
LONG ANSWER
Determine the measure of ےECB, ےBDC, ےBAD, and ےDBE, where E is the centre of the circle.
So first of all we always start with a table that has STATEMENT on one side and REASON on the other.
It's obvious at this point that BE and CE are radii, therefor triangle BCE is an isosceles triangle. This also means that ےEBC and ے BCE are the same.
We now know the exact amount of ےBCD and according to cyclic quadrilateral theorem ے BCD's and ےBAD's sum should equal 180°. So in order to find ےBAD, we subtract 30° and 40° from 180° and come up with 110°. Therefore ےBAD is 110°.
Another thing we looked at were the two triangles that are subtended by the same arc; triangle BCE and triangle BCD. Since all of us know of the central inscribed angle theorem ( AKA the star trek theorem ) we can easily point out that ےBDC is half the amount of ےBEC. As a result ےBDC = 100°/2 which equals 50°.
We only have one angle left! Angle DBE! So if we look closely at triangle BCD you'll realize that we only have one angle left to find, ےDBE. We also know that every the angles of a triangle sum up to be 180° and by subtracting every angle that we have we'll be able to come up with the value of ےDBE! :D
Which in this case in 20°! =)
Once you've found your information and proofs, your table (including "Statement" and "reason") should look something like this:
MULTIPLE CHOICE
In the diagram below, R, S, T, U are points on the circle with RS = RU = UT and SU = ST. Find the measure of ےUST.
a) 31°
b) 35°
c) 36°
d) 40°
e) none of these
The first thing you should always do is mark down what is given to you. Have a wishful thinking colour, a colour used for given facts and a colour used for things you have proven! ( it comes in handy :D )
I'll use purple as the facts that are given, blue for what we're looking for and orange for the facts i prove. =)
There's no particular order to do this in, but what i looked at first was the isosceles triangles. We can easily point out that ےRSU and ےRUS are the same and ےSUT = ےSTU.
Now if we look at RS, RU and UT they are all the same length. Which means that the angles subtended by this chord length will all be the same. SOOO.. ےRST = ےRUT = ےUST.
In order to find the exact degree that angle is we make three equations. (this is like going back to substitution in linear equations). Here are the three equations (let ےSRT = w):
1. w + z = 180
2. 3y + z = 180 --> z = 180 - 3y
3. w + 2y = 180 --> w = 180 - 2y
If we substitude 2 and 3 into 1, we can find out what Y is, which is ےUST.
180 - 2y + 180 - 3y = 180
180 + 180 - 180 = 2y + 3y
180 = 5y
36 = y
And there you have it!
c) 36° is the correct answer. =)
That's all for tonight! Sorry for the late entry, i had to go and watch my brother's christmas concert! haha. =) Well, no homework tonight, just study! Test tomorrow. Good luck guys!
HAPPY HOLIDAYS! =]
And the next scribe will bee..... -Zeph! Enjoy. =)
Blogging on blogging
Well the circle geometry was pretty fun till we had to start proving what was true, and that I think was the only thing in this unit that is still a little fuzzy. I tried my hardest on this unit but I really need to find a trick to memorize that darn theories. This unit I think was definitely one of the better ones, but good luck to all on the test.
B0B Natnael
Well, this unit as Cherieeee said was quite easy, and the thing that made it easy, i think, is that its pretty straight forward. I mean, right now we now whats on the test (at least the layout).
I think everyone will do very good on this test...and some excellent(its not u Lauressa..jk)
goodluck on the test everyone! ;D
I think everyone will do very good on this test...and some excellent(its not u Lauressa..jk)
goodluck on the test everyone! ;D
BEEE - OHHH - BEEE (.. spells BOB)
Ahhhhhh! Another unit test!
Oh well, nothing to worry about. I honestly think that this unit, for me, was easier to understand than the ones we've had in the past. There's just something about circles, triangles and geometry that make them easy to learn. There were times when I hated this unit because of all the PROVING we needed to do. But I guess, the more proving we did in class, the easier it seemed, and my hatred lessened. Today we had the pretest in class, and I don't think I did my best. Although, I did put in my first effort, and got about 70% of the questions right! "Sweeeeeet!" Anyway, good luck to everyone on the test tomorrow!
-- Cherrrieeeee
Oh well, nothing to worry about. I honestly think that this unit, for me, was easier to understand than the ones we've had in the past. There's just something about circles, triangles and geometry that make them easy to learn. There were times when I hated this unit because of all the PROVING we needed to do. But I guess, the more proving we did in class, the easier it seemed, and my hatred lessened. Today we had the pretest in class, and I don't think I did my best. Although, I did put in my first effort, and got about 70% of the questions right! "Sweeeeeet!" Anyway, good luck to everyone on the test tomorrow!
-- Cherrrieeeee
December 13, 2006
Test Prep Exercises - Questions 1, 2 and 8
hey guys. Sandy and I had an agreement to switch days, if she chose me tomorrow.
So yes, its me EEDCE.
(1) In the diagram below, AB and CB are tangents to a circle with radius 10. If angle AB find x, the shortest distance from B to the circle.
(2) Given: Circle with centre C
- Angle 1 = Angle 2
Prove AB=CD
Statement Proof
AD // BC given
EA is tangent at A given
Angle 8 = Angle 7 Tangent-Chord Theorem
Angle 7 = Angle 5 Alternate Angles
Angle 6 = Angle 8 - Angle 9 Tangent-Chord Theorem
Angle 1 = Angle 2 Triangle ABC ~ Triangle AEC
Okay. thats it folks.
homework is to finish the rest of this pre-test if not yet done so.
SANDY will take it away tomorrow.
So yes, its me EEDCE.
(1) In the diagram below, AB and CB are tangents to a circle with radius 10. If angle AB find x, the shortest distance from B to the circle.
cos50°=10/h
h = 10/cos50
h = 15.557units
x = h - 10
x = 15.557 - 10
x = 5.557 units
(2) Given: Circle with centre C
- Angle 1 = Angle 2
Prove AB=CD
statement proof
Angle 1 = Angle B given
BC ~= CD ~= AC radii
Triangle ABC is isoscles from above
Triangle ACD is isoscles from above
Therefore, AB cannot be congruent to CD.
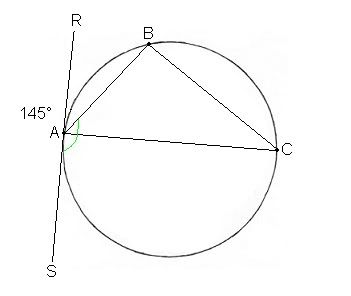
8. Complete the proof:
Diagram clarification: E, D, C are collinear
Given: EA is tangent to the circle at A
AB EC
Statement Proof
AD // BC given
EA is tangent at A given
Angle 8 = Angle 7 Tangent-Chord Theorem
Angle 7 = Angle 5 Alternate Angles
Angle 6 = Angle 8 - Angle 9 Tangent-Chord Theorem
Angle 1 = Angle 2 Triangle ABC ~ Triangle AEC
Okay. thats it folks.
homework is to finish the rest of this pre-test if not yet done so.
SANDY will take it away tomorrow.
Circle geometry
Salutations fellow math students,
Today, for all you naughty kids that skipped (haha jk), we worked on five different circle problems that Mr. K had put up for the class to do. They were:
1) A chord that is 10cm long is 12 cm from the centre of a circle. Find the radius.
2) Line AB and line CD are two parallel chords in a circle. Line AB is 10cm and Line CD is 14 cm. Find the radius if the chords are 3cm apart.
3. a) O is the centre of each circle. Find the measures of the indicated angles. Justify your answer.
3. b)
4) Given:
-Line AC is tangent at B
- Angle 1 is congruent to angle 2
Prove: Line BD // Line FC
So yeah that's what happened in class. Homework was posted up on the blog by our very own math teacher, Mr. K. And I choose........................................................................................SANDY cheeks to be our scribe for tomorrow's class.
Today, for all you naughty kids that skipped (haha jk), we worked on five different circle problems that Mr. K had put up for the class to do. They were:
1) A chord that is 10cm long is 12 cm from the centre of a circle. Find the radius.
2) Line AB and line CD are two parallel chords in a circle. Line AB is 10cm and Line CD is 14 cm. Find the radius if the chords are 3cm apart.
3. a) O is the centre of each circle. Find the measures of the indicated angles. Justify your answer.
3. b)
4) Given:
-Line AC is tangent at B
- Angle 1 is congruent to angle 2
Prove: Line BD // Line FC
So yeah that's what happened in class. Homework was posted up on the blog by our very own math teacher, Mr. K. And I choose........................................................................................SANDY cheeks to be our scribe for tomorrow's class.
December 12, 2006
BOB for the Circle Geometry Test
Hi everybody and in a few days it will be our circle geometry test and I'm almost ready. All I need to do is to study and get down all the stuff I miss about 2-3 weeks ago. I need to practice the recent work which is to prove somthing using the given info in a question which is not that bad but since it's new it doesnt hurt to do stuff. I need to get my theorems straight and finish the new review. That's all and good luck on the pre-test and test. =)
Circle Geometry Test Practice
As promised, here are 8 problems to help you get ready for the test.
Remember, as I said in class, if you start working on one of these questions and get the horrible feeling in the pit of your stomach ... STOP. Go on to the next problem and try it out. If that happens with all 8 problems take a break and come back to them later ... lather. Rinse. Repeat. ;-)
We'll go over all the questions in class tomorrow.
Remember, as I said in class, if you start working on one of these questions and get the horrible feeling in the pit of your stomach ... STOP. Go on to the next problem and try it out. If that happens with all 8 problems take a break and come back to them later ... lather. Rinse. Repeat. ;-)
We'll go over all the questions in class tomorrow.
December 11, 2006
180.bmp)
December 10, 2006
Did you know?
Did you know I can see your classroom from two windows?!
My first window is your blog. I am excited by what I see and hear! I never cease to be amazed by the quality and sophistication of your scribes; you constantly achieve new heights in illustrating and annotating your scribes. More than that I am so impressed when you celebrate each others’ learning, when you are creative, and when you critically reflect upon your learning in your BOBs.
Did you know Mr. K’s blog is my second window? I admire and respect what I see and hear here too! Did you know that Mr. K celebrates your learning on his blog? that he reflects upon what best helped you to learn and why? that he unselfishly shares all he knows with those who read his blog? that he learns from the conversations on his blog? that he writes with passion and is creative? and that he commits many random acts of kindness by honoring other teachers’ accomplishments in his posts? Did you know all he expects of you, he shares those same expectations for himself?
Did you know that because of all that and more, Mr. K.’s blog has been nominated for “Best Teacher Blog 2006” on the Edublog Awards website?
I just happen to think that no one deserves this honor more than Mr. K.
What about you?
December 06, 2006
Circle Geometry - Investigation 8
Good evening everyone. Today Mr. K talked to us about our flickr photo assignment at the beginning of class. The due date is now on Monday. Just a little reminder for you all.
We did investigation 8 today. Here were the instructions.
1.
(a) Construct a chord AB in the circle.
(b) Construct a tangent, RS, to the Circle at A. The tangent should not be perpendicular to AB.
(c) Measure the angle of RAB.
You can plot down the points anywhere you'd like. Just be sure that your chord and the tangent line aren't perpendicular. I measured angle RAB and ended up with 35° with mine.
(d) Construct a point, C, on the circle, on the opposite side of chord AB from angle RAB.
Some of us where have a few problems plotting down C. We did plot C opposite of the chord AB, but had it plotted down inside the circle. The question asks to plot it on the circle, meaning on the circumference. What we did was plot it down in the circle which was different from what was being asked. So, just look out for those small things and read the question carefully.
(e) Construct and measure inscribed angle ABC. What do you notice?
From looking at the slide below, you will notice that angle ACB is the same as RAB.
(f) Measure angle SAB.
As you can see, angle SAB measures 145°.
(g) Construct a point, D, on the circle, on the opposite side of the chord AB from angle SAB.
(h) Construct and measure inscribed angle ADB. What do you notice?
Angle SAB and ADB are the same.
Here is sort of a final drawing on all the angles that were measured.
THIS HERE IS CALLED THE TANGENT-CHORD THEOREM.
(i) Can you articulate a general rule for what you have found? Your sentence should begin:
"If an angle is formed between a tangent line and a chord, then...."
The rule here is simply that "If an angle is formed between a tangent line and a chord, then the inscribed angle subtended by the opposite side of the chord is congruent."
We did investigation 8 today. Here were the instructions.
1.
(a) Construct a chord AB in the circle.
(b) Construct a tangent, RS, to the Circle at A. The tangent should not be perpendicular to AB.
(c) Measure the angle of RAB.
You can plot down the points anywhere you'd like. Just be sure that your chord and the tangent line aren't perpendicular. I measured angle RAB and ended up with 35° with mine.
(d) Construct a point, C, on the circle, on the opposite side of chord AB from angle RAB.
Some of us where have a few problems plotting down C. We did plot C opposite of the chord AB, but had it plotted down inside the circle. The question asks to plot it on the circle, meaning on the circumference. What we did was plot it down in the circle which was different from what was being asked. So, just look out for those small things and read the question carefully.
(e) Construct and measure inscribed angle ABC. What do you notice?
From looking at the slide below, you will notice that angle ACB is the same as RAB.
(f) Measure angle SAB.
As you can see, angle SAB measures 145°.
(g) Construct a point, D, on the circle, on the opposite side of the chord AB from angle SAB.
(h) Construct and measure inscribed angle ADB. What do you notice?
Angle SAB and ADB are the same.
Here is sort of a final drawing on all the angles that were measured.
THIS HERE IS CALLED THE TANGENT-CHORD THEOREM.
(i) Can you articulate a general rule for what you have found? Your sentence should begin:
"If an angle is formed between a tangent line and a chord, then...."
The rule here is simply that "If an angle is formed between a tangent line and a chord, then the inscribed angle subtended by the opposite side of the chord is congruent."
RULE
IF AN ANGLE IS FORMED BETWEEN A TANGENT LINE AND A CHORD, THEN THE INSCRIBED ANGLE IS SUBTENDED BY THE OPPOSITE SIDE OF THE CHORD IS CONGRUENT.
IF AN ANGLE IS FORMED BETWEEN A TANGENT LINE AND A CHORD, THEN THE INSCRIBED ANGLE IS SUBTENDED BY THE OPPOSITE SIDE OF THE CHORD IS CONGRUENT.
And yes, I did mean to repeat that, and repeat it in a way that will be easily read. It's so that you can remember the rule better. Through repetition. Hopefully it works.
The HOMEWORK for tonight is to Re-do the questions we had trouble with in EX. 33. And also EX. 34.
REMINDER: There will be a quiz maybe tomorrow, or the next day after.
I chose the next scribe to be Natnael.
Good Night~
The HOMEWORK for tonight is to Re-do the questions we had trouble with in EX. 33. And also EX. 34.
REMINDER: There will be a quiz maybe tomorrow, or the next day after.
I chose the next scribe to be Natnael.
Good Night~
December 04, 2006
Today's class we started off by handing in our projects. I hope everyone gets over 80% just like Mr. K said we should.
After that he showed us some examples of the 40s student's trigonometry pictures, so have a better clue of what to do with our quadratic function pictures. Make sure it has a lot of details a quadratic function should have.
*Reminder it should be uploaded on flickr by Thursday.
We went over some question in our exercise #32 which were # 10, 12, 13
#10 was a question that didn't really fit in with any unit we did so far but it was a fun question to solve.
#12 this question we had to find the ticket price that will yield maximum profit.
This is a good review for our Analytic Geometry unit.
revenue = (# ticket) (cost/ticket)
let x = 1 discount of $3.00
R (x) = (1000 + 100x) (60 - 3x)
roots @ x= -10 x= 20
We ended up with the price $45 per ticket.
#13
A) y= x and y = -1/2x+2
B) x=-1/2x+2
2x=x+4
3x=4
x=4/3
(4/3, 4/3)
C) 8/3
After going over some homework we started on our Investigation #7
This album is powered by
BubbleShare
- Add to my blog
"If two tangents are drawn from a common point, exterior to a circle, then...the tangent lines are the same measure"
"If a radius intersects a tangent line, then...the angle is 90 degrees"
After all of that the next step is to go back and construct a new point S exterior to the circle and repeat steps b, c and d for point S.
Today's homework is exercise #33
Make sure to attempt every question. The ones you are really stuck on should be looked at in class.
And most of you may notice that the scribe list is now on our fourth cycle!! that's a lot of scribing!. I just wanted to say I'm really proud of every one's work. Look how far we came with our scribing, we're using a lot more tools to make our post explained to the best of our abilities.
Okay enough of that...the next scribe for Wednesday (Tuesday afternoon NO SCHOOL!) is... MERIAN!
GOOD NIGHT!
After that he showed us some examples of the 40s student's trigonometry pictures, so have a better clue of what to do with our quadratic function pictures. Make sure it has a lot of details a quadratic function should have.
*Reminder it should be uploaded on flickr by Thursday.
We went over some question in our exercise #32 which were # 10, 12, 13
#10 was a question that didn't really fit in with any unit we did so far but it was a fun question to solve.
#12 this question we had to find the ticket price that will yield maximum profit.
This is a good review for our Analytic Geometry unit.
revenue = (# ticket) (cost/ticket)
let x = 1 discount of $3.00
R (x) = (1000 + 100x) (60 - 3x)
roots @ x= -10 x= 20
We ended up with the price $45 per ticket.
#13
A) y= x and y = -1/2x+2
B) x=-1/2x+2
2x=x+4
3x=4
x=4/3
(4/3, 4/3)
C) 8/3
After going over some homework we started on our Investigation #7
This album is powered by
BubbleShare
- Add to my blog
"If two tangents are drawn from a common point, exterior to a circle, then...the tangent lines are the same measure"
"If a radius intersects a tangent line, then...the angle is 90 degrees"
After all of that the next step is to go back and construct a new point S exterior to the circle and repeat steps b, c and d for point S.
Today's homework is exercise #33
Make sure to attempt every question. The ones you are really stuck on should be looked at in class.
And most of you may notice that the scribe list is now on our fourth cycle!! that's a lot of scribing!. I just wanted to say I'm really proud of every one's work. Look how far we came with our scribing, we're using a lot more tools to make our post explained to the best of our abilities.
Okay enough of that...the next scribe for Wednesday (Tuesday afternoon NO SCHOOL!) is... MERIAN!
GOOD NIGHT!
December 01, 2006
Circle Geometry!
To start off today's class we all congratulated Crysta on her Fantastic! scribe post. Once again, great job Crysta! Mr. K was also trying to explain to us the meaning of how much one million (1000000) really is. If we broke it down to how long it would take to reach one million by receiving one penny each second. When we finished calculated it, it took 11.5 hours. Then we decided to calculate how long it would take to reach a billion (1000000000). We all found out that it would take 32 years to reach that. We also realized that we haven't even lived for a billion seconds yet AND one billion penny's will fit into 5 school buses. Quite interesting, don't you think? If you're wondering how Mr. K knew all this, he went to this website
http://www.kokogiak.com/megapenny/
Anyway, once we got back onto to track we discussed questions that we had trouble with from last nights homework and we did Investigation 5.
(a) Choose 6 different points on the circumference of the circle. Do not space them out evenly. Label these points A, B, C, D, E and F.
So this part is pretty straight forward. We start with a blank circle and add 6 points anywhere on the circumference.
This album is powered by BubbleShare - Add to my blog
(b) Construct angles ACB, ADB and AEB.
For this part of the investigation, we're pretty much making chords, but these are to make angles.
This album is powered by BubbleShare - Add to my blog
(c) Measure the angles formed at C, D and E.
So here we take the angles and measure them. It's best to write them down that way we can keep track of them. instead of just adding it to the picture.
This album is powered by BubbleShare - Add to my blog
When I completed this step in class, i got this:
ACB = 13°
ADB = 13°
AEB = 13°
Notice a pattern?
(d) Construct angles EAF, EBF and ECF.
Here, we will repeat what we did for (b).
This album is powered by BubbleShare - Add to my blog
(e) Measure the angles formed at A, B and C.
Here we'll do the same as (c).
EAF = 60°
EBF =60°
ECF =60°
(f) What do you notive about all these angles? Can you articulate a general rule for what you have found? Your sentence should begin:
"If two (or more) inscribed angles are subtended by the same arc, then..."
Have you seen the pattern to these? Well, If you have you've noticed that all the angles come out to equal the same. To complete the sentence can be said in different ways.
i) ... they have the same measure.
ii) ... are congruent
iii) or in your own words!
So there you have it! investigation 5! I don't know if it's just me but these things are super fun to do. haha. (:
Anyway. Homework for the weekend is:
1. Attempt to complete Investigation 6. (but if you get frustrated with it, then leave it be)
2. Exercise 31.
3. Project (due monday)
4. Picture of a parabola put onto Flickr. (due thursday)
REMEMBER TO DO YOUR HOMEWORK, GUYS. =)
As for the next scribe.. i think** the only person left is MELISSA! .. haha. if i'm wrong, correct me, otherwise, Have a great weekend!
Subscribe to:
Posts (Atom)
