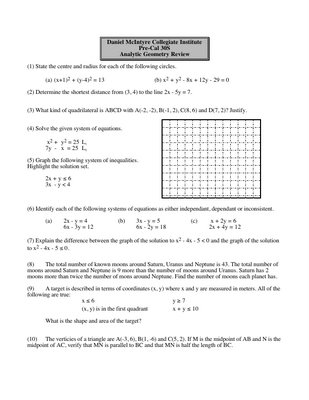
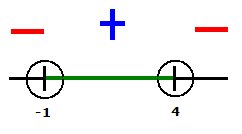
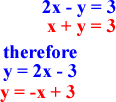Okay so i didn't get to do my post when i was scribe last time cause my net wasn't being very nice. Today in class we did investigations 3 and 4.

Investigation 3:
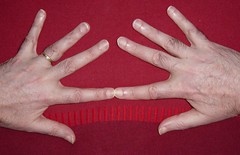
1.(a) Use what you know about chords to find the centre of the circle. Erase all construction lines once you're done.

This is how you do question 1.(a) (If you do have any questions, this question is explained in the previous scribe):

This album is powered by
BubbleShare
- Add to my blog

(b)Construct diameter AB.

Heres what you do:

This album is powered by
BubbleShare
- Add to my blog

(c)Choose 4 different points on the circumference of the circle. Do not space them out evenly. Label these points C, D, E and F.

This questions is self-explanatory:

This album is powered by
BubbleShare
- Add to my blog

(d)Construct chords AC and BC.
(e)Measure the angle formed at C

Draw lines from A to C and from B to C and measure the angle C:

This album is powered by
BubbleShare
- Add to my blog

(f) Repeat steps (c) and (d) for the points labeled D, E and F.

It's pretty straight forward:

This album is powered by
BubbleShare
- Add to my blog

(g) What do you notice about all these angles? Can you articulate a general rule for what you have found? Your sentence should begin:
"If an inscribed angle is subtended by a diameter then...."
If an inscribed angle is subtended by a diameter then.... the inscribed angle is always 90°.

So that was Investigation 3. We started Investigation 4 but did not get a chance to go over it in class. THE SCRIBE FOR THE WEEKEND IS .. SANDY !

Dictionary

For those of us who missed out on our math dictionary I'll fill you in.
Well it's actually just the end of what we couldn't finish yesterday.

• Being able to find the root sum and root product of f(x)=2x^2-6x+7

A=2 Root sum= -b/a Root product= c/a
B=-6
C=7 -(-6)/2 = 3 7/2

• Find a quadratic function whose sum is -3 and whose product is 5/2
  
  Root sum= -b/a Root product= c/a
  -3/1 = -b/a 5/2= c/a
  
  -6/2 = -b/a both must have a common denominator 5/2= c/a
  

a=2 f(x)=2x^2+6x+5
b=6
c=5

Circle Geometry

Well where should I start. In class we were given our first assignment in class, it wasn't hard but it was relatively new to some of us. Investigation 1 and 2 were our given assignments and here are completed ones in case anyone didn't quite get it .

INVESTIGATION 1
A- Construct 4 different chords (a straight line joining two points on the circumference of a circle), AB ,CD, EF, and GH

• But none of them should be diameters (longest possible chord in a circle that passes through the center) nor should they be parallel. The 4 different chords are in black
  

B- O is the center of the circle. Construct lines from the center that are perpendicular to each of the chords. AB, CD, EF and GH. Label the new points where the new lines meets W, X, Y and Z , The perpendicular lines will be in red . The perpendicular lines are also known as bisectors, because they cut the chord into two.

C- Measure the lengths of the AW and BW. Do so with the rest so every chord has two measurements on either side of the perpendicular line

D- Now after your picture is drawn you should see a relationship between a chord and their perpendicular line.

A LITTLE HELP: "A line perpendicular to a chord, that passes through the center of a circle, the chord."

Here is an example of what your drawing should somewhat look like

INVESTIGATION 2
A- Like the A in Investigation 1 construct 4 different chords AB, CD, EF and GH

• Remember none of them should be parallel to each other nor be diameters
  

B- Construct perpendicular bisectors for each chord. Each bisector should be a chord.

KEY: the way to find the bisector is to measure the chord, and find the midpoint. At that midpoint line up a triangle from your geometry set to make a 90 degree angle and draw the perpendicular.

C- What do all the bisectors have in common? Well once your done they should all intersect at the same point.
D- What can you say about any perpendicular? You could say that the perpendicular bisector of any chord will always dissect the center of the circle.

This diagram should give you an idea of how it should look


Example: chord AB. Length of chord divided by two = point where bisector should cross

To complement our learning we also learned some definitions to go along with our new unit
Bisect- to cut into two exact equal halves
Subtend(subtended)- to hold arms of an angle open by an arc

Well that's it thats all folks
Oh yea before I forget

Share the kindness and leave some kind comments on our friends blog in Scotland
If you forgot the address its exc-el.org.uk/blogs/s3srcibeposts, I checked it out and its pretty neat!


Ex 30 for homework.
NEXT will be ......................Crysta!

Working Harder or More Effectively?

Greetings Pre-Cal 30S! From your BOBs, it appears you are really working hard and feeling that to be more successful, you need to "practice more", "study more", and "need more practice". My sense is you may be practicing and studying now; I wonder if you think you could be more effective in your efforts? I know from lots of experience (60 years worth) that habits can ensure my reaching my goals or not. And often, I've studied more, and practice, but not really effectively. Given that, I think I've found 7 habits, that if I've worked at them, have helped me be more effective! Have you seen these 3? Do you think these habits can be of value to you too? Or are these a habit for you already?

--------------------------------------------

I think this short autobiography is a good introduction to Habit 1.

There's A Hole In My Sidewalk: AUTOBIOGRAPHY IN FIVE SHORT CHAPTERS

by Portia Nelson
Chapter 1
I walk down the street.
There is a deep hole in the sidewalk.
I fall in.
I am lost.... I am hopeless.
It isn't my fault.
It takes forever to find a way out.
Chapter 2
I walk down the same street.
There is a deep hole in the sidewalk.
I pretend I don't see it.
I fall in again.
I can't believe I am in the same place.
But, it isn't my fault.
It still takes a long time to get out.
Chapter 3
I walk down the same street.
There is a deep hole in the sidewalk.
I see it is there.
I still fall in.... It's a habit.
My eyes are open.
I know where I am.
It is my fault.
I get out immediately.
Chapter 4
I walk down the same street.
There is a deep hole in the sidewalk.
I walk around it.
Chapter 5
I walk down another street.

Habit 1: Be Proactive®
Take responsibility for your life.

--------------------------------------------------------------

Does this cartoon say it all for Habit 2?

Habit 2: Begin with the end in mind.®
Define your mission and goals in life..

-----------------------------------------

Finally, Habit 3: Rocks, Pebbles, Sand, Water—And Calculus is which?

A time management specialist was asked to give a presentation on her specialty. She decided to do a demonstration. First she asked her assistants to bring a big bucket and put it on the table in front of the audience. Then she asked for large, grapefruit-sized rocks and filled the bucket with them.
"Is the bucket full," she asked?
"Yes!" said the crowd, but she asked for more to put in anyway. This time her assistants brought in pebbles. She poured the pebbles in the bucket and it held a surprising number in the space between the big rocks.
"Now is the bucket full?" she asked.
"Yes!" "No!" "Yes!" "No!" said various persons in the crowd. Some people were uncertain; some were getting suspicious. The time management specialist asked for more. This time the assistants brought her sand. She poured sand in the bucket and it filled the spaces between the pebbles.
"Now is the bucket full?" she asked.
"No!" they answered. By now, everyone was suspicious. So she asked for water and poured in quite a lot. Now no one could think of anything else that could fit in that bucket.
"What does this process demonstrate?" asked the time management specialist.
One member of the audience spoke up: "No matter how busy you are, you can always fit in one more thing."
"I can see how you might think that was my point, but it is not," said the specialist. "I was trying to show you that if you don't put the big rocks in first, you'll never get them in at all!"

Habit 3: Put First Things First®
Prioritize and do the most important things first.

What do you think?
Best,
Lani

And by the way, these come from 7 Habits of Highly Effective Teens by Sean Covey.

Zeph's Notebook/Dictionary: Algebra

just to make things a bit organized, this scribe post is divided into two post. one section will be the circle geometry notes we took and the other post will be the algebra notes. this is so that this scribe post doesnt end up having the labels, circle geometry and algebra, at the same time.

Note: there is still more algebra notes to come from the next scribe!


REMINDERS:

~ FLICKR/NUMBER ASSIGNMENT - GET MORE PICTURES!! TOTAL OF 5 ALTOGETHER
~ "LOST IN MATHLAND" ASSIGNMENT DUE THE DAY AFTER TOMORROW!!
~ NO HOMEWORK
~ LAURESSA'S SCRIBE
~ GEOMETRY SET

Zeph's Notebook/Dictionary: Circle Geometry

just to make things a bit organized, this scribe post is divided into two post. one section will be the circle geometry notes we took and the other post will be the algebra notes. this is so that this scribe post doesnt end up having the labels, circle geometry and algebra, at the same time.


CIRCLE GEOMETRY

Note that the small "o" stands for "degrees" not to be confused with exponent zero...





REMINDERS:

~ FLICKR/NUMBER ASSIGNMENT - GET MORE PICTURES!! TOTAL OF 5 ALTOGETHER
~ "LOST IN MATHLAND" ASSIGNMENT DUE THE DAY AFTER TOMORROW!!
~ NO HOMEWORK
~ LAURESSA'S SCRIBE
~ GEOMETRY SET

Setting A Circular Mood ...

We began our study of circle geometry today. I thought this might be a good way to "set the mood" for this next unit of study... ;-)

And another test finished!

And yet another test accomplished!
Crysta set me to scribe on Thursday/Friday, but there was just a pre-test.

HOWEVER,
Today was the day we had our Analytic Geometry unit test. How do you think you guys did? I'm positive to say I did my best on the whole test, except my mind fell apart on the last two questions :

Anyway,
There isn't much to write for today's class, but I sure hope everyone did well on it, despite the difficulties of the questions.

A few reminders:
1. FLICKR ASSIGNMENT -
In class we discussed the assignments, and the pictures involved with it. According to Mr. K, Flickr will not 'activate' (for lack of a better word) our accounts unless we have a minimum of five (5) pictures. So remember to take four more pictures (not of anyone's faces, thanks), of random things, and upload it into your flickr account.

2. MATH PROJECT #1
Don't procrastinate! Get started on your math project now! Reminder: Due Friday, December 1^st, 2006. (Oh wow, DECEMBER ALREADY?!?!?!) You have a choice of working by yourself, or with a partner. No outside help, but from books, the internet, or Mr. K! AND also remember that the minimum and expected mark we should all get is an 88%. Let's make them good!

I hope this is a good scribe for today's class, and helps people in reminders. I choose the next scribe to be ....

" -Zeph "

Late BOB

Hey guys,
well, this unit, i guess, have been tough on you guys, and I really hope u guys be fine on Monday. But for me, this unit, have been really easy, and I think I'm doing fine in this unit. One of the reasons, I think, is that at first I tried to get the concept...and i was so interested in the 'dimension' stuff. So, I tried to get the main idea, and once i got it...i dunno, but it wasn't that hard.

good night

BOB - Analytic Geometry

I found this unit to be quite fun with some challenging points to it too. Like people have mentioned already on their BOB's, I tend to make the smallest errors which really screws things up. I hope I'll be aware of the mistakes I make on the test so that I could correct them. I think I'll need to do more reviewing as well so that I can really get the concept down.

BOB - Analytic Geometry

Well, for myself I found this unit of "Analytic Geometry" much like a bad shopping trip. There you are, searching and searching for the perfect outfit, but no success. Although it only takes awhile to enter that one store and see that perfect top you've been looking for hanging on display. Finding that perfect top would be when you finally find the equation(s) thats being asked to solve. Understanding what the questions being asked for is the hard part, but once you get that resolved and figuire out the equation(s).. its a breeze from there, much how its a breeze to just pick up the top and walk to the cashier. Although I realized you have to be very aware while solving. Be aware of little errors you can make which will screw the whole process up. Much like shopping, be aware of the price and be sure you can actually afford the item.
- Other then that. GOOD LUCK AND GOOD NIGHT.

don't mind my metaphoric BOB entry, we just finished poetry in english =) Haha.

BOB!

Another unit done. Analytic Geometry was a hard, yet fun unit. The distance between a circle and a line, I'm pretty sure I have that down. I'm still having trouble with the linear equations. The word problems are difficult also, but I always seem to mess up on the smallest things. Well this weekend I'll be studying for this test. I need to practice more.

'so good luck on the test! :)

BOB v.4 An Analytical Analysis

well that was weird... I had to re-register back to blogger.com but anyways i got my account back and i'm still registered at this blog. so thats good news for me!

i wont be coming to school on monday cuz i found out that i have a viral infection. all the clinics were closed and my appointment was scheduled til the second week of december. i'd probably be dead by then. haha. anyways, all the clinics that my parents suggested on me going to were all booked and on friday, my parents sent me to the hospital. there were so many people at the hospital. you have to die to get your way in...literally. what i meant is that there were so many patients waiting in line that one of them already died. the paramedics were there and the mother was yelling "I WANT MY SON BACK!" and was freaking out. Well i was very lucky because we found a clinic near the hospital that wasnt all booked and the doctor said that i have a viral infection. the medicine that he prescribed to me had strange side-effects such as nausea, headaches, bad dreams, and excessive sleep & sleepiness. b4 u no it, i'll be back at school and i'll be alrite.

in analytic geometry, word problems are still an obstacle thats just in the way, but once i find the equation everything seems to be straightforward. solving the system of equations are easy for me but one mistake anywhere in the long process can equal death. graphing the equations is the topic that i needed to focus more on and other than that, analytic geometry seemed ok.

~ zeph

BOB

Okay, so this is my BOB for the unit analytic geometry. I think out of all units this is the one that i found the hardest. I missed one day of class and i was so confused for the following classes. I think I've finally gotten the hang of it and realize what is asked in the question. I'll just have to study some more over this weekend to be completely ready for the test on Monday. Other than that, i think everything is okay.

Hope you guys are having a great long weekend! =)

BOB for the Analytic Geometry Test

Hi everyone and this is my BOB. I get some of the stuff on the unit of analytic geometry but I need a bit more practice especially on the inequality questions. I seem to screw up a lot so I'm guessing I need a bit more work. I'll just study to get the other stuff into my head. About the multiplying with the fingers blog was cool. It works and it's kind of fun doing it also.

It's All In Your Hands

This picture shows that 7 x 7 = 49 ... click on it to find out how. ;-)

Don't forget about your flickr assignment.

Cheers!
Mr. K.

blogging on blobbing

I think that this unit about analytic geometry is difficult for me. This unit started off really easy with all the equation of the circle and distance between a point and a line. Then it got a little bit confusing with the systems of linear equation with three unknowns and word problems. I think that I'm average at everything but struggles at doing systems of linear equation with three unknowns and word problems. I wish that we can do a little bit more of word problems. I hope that everyone do well for the test on Monday. That's it for my BOB.

B-O-B SPELLS BOB!

So another unit done, and another test to come. (Ooh, that rhymed!).
In this unit, I was struggling quite a bit in the beginning. Finding distance between two points is simple, but sometimes when it comes to distance from a line to a point, depending on the question, I have a little difficulty. However, I have been working on review questions from past exercises and I think I finally got the hang of it! Well we won't know for sure until the test! Aw, and I miss the pretest today, because of jazzfest : (. But I hope everyone does well on this pretest! See ya guys on Thursday!
♥ ♥ Cherrieeeee

B-B-B-O-B

The unit went by pretty quick. I understand the point to line stuff. I had little difficulty, the only thing that messes me up sometimes would be the number line because I forget what I'm looking for and if its true or false. That is all i have to say about this unit. I hope everyone does well!

-SAMUS

Hey everyone, I'll be scribing for both today and yesterday's class.

Yesterday we had a what seemed like a shorter class, because Mr. K had to leave early.

In the beginning of class Mr. K talked about a new project we should start on. Which involves our favorite number, and why it's so special.

* Dont forget to sign up to flickr.com so we can post up our pictures to the tag PC30SF06

We had a review sheet that day on "Quadratic & Rational Inequalities"

Some of us had a hard time solving the questions, so Sam found our good friend Mr.Clark. He came and helped us with question 1 (h).

2x² -7x +3 >= 0
(2x-1)(x-3) >= 0
e.p. (3,1/2)

Solution
(-oo, 1/2] U [3, oo)


He also did another example

(x+2) (x-1) / x+3 <= 0
e.p. (-2,1,-3) Nothing else really happened in that class so we continued to work on our review sheet.

~~~~~~~

Today's class we worked on the question Mr K gave us.
Which were: Slove
1. a) x²+3x >= 18
b) 2x+5/x+1 <= x+1/x-1
c) |3-4x| > 9
d) |5x-8| <= 2

2. There are $950 in $5, $10, and $20 bills in the cash regester. There are a total of 71 bills. The number of $20 bills is 7 less than the total number of $5 and $10 bills. How many bills of each denomination are in the cash regester.

We had time to work on them and corrected it with the class.

a) x²+3x >= 18
x=3x-18 >= 0
(x-3)(x+6) >= 0


Solution
(-oo,-6] U [3,oo)

b) 2x+5/x+1 <= x+1/x-1
x can not = -1 , 1
we multiply each side by (x+1) (x-1) then we'll get.
2x² + 3x-5 <= x²+2x +1
x² + x -6 <= 0 (x+3) (x+2) <= 0

Solution
[-3, -1) U (-1, 1) U (1, 2]

c) |3-4x| > 9



d) |5x-8| <= 2


2) # 5 bills = F
# 10 bills = N
# 20 bills = W

equations
F+ N+ W = 71
5F + 10N + 20W = 925
-F - N + W = -7





There are 21 $5 bills, 18 $10 bills and 32 $20 bills.

Don't forget our pre test is tomorrow and our test for this unit will be on Monday.

Tomorrows scribe isss........ Crysta!! have fun!!

** Don't forget to do the review down there\/

Analytic Geometry Review and Reminders ...

Your first project was handed out in class today. It is due next Friday, December 1. You can use any presentation format you like. Mr. Harbeck and I have started collecting a list of free online tools you can use any time you have a project to do. You can find it here.

Don't forget your first flickr assignments are due on Friday. (Take a picture of an "interesting" (to you) number using your cell phone or digital camera. Upload it to flickr. Include a description of why you chose that number and tag it pc30sf06.)

Below is the review sheet we talked about in class today ... try to finish it for class tomorrow. The test is next Monday.

Solving Inequalitie Equtions & A Splash of Review

Well well well. Another friday scibe post!
Okay. So lets get this scribe going.

Minutes after the 5th period bell, we all walk into our Pre-Cal class, as we do everyday and by our dismay we spot Mr. K holding a small stalk of papers. Since we're all "smarter then the average bear", we immediately knew a lovely quiz was coming in our direction. So after a brief discussion of wither or not we should include quizes for marks, we all got to work. The quiz was nothing new; a nicely summed up quiz about everything we learned so far in 'Analytic Geomtry'.

After self marking our peer's quizes, Mr. K placed the following questions on the board.

S O L V E:

Question 1:
x²+x<6

Okay, so we haven't seen much of these before and you may be a little puzzled.
BUT- think back to the Mathmatician who set the house on fire
"Turn the problem into something we already know how to solve"

First and foremost- Ignore the "<" for now, think of it as an "="
x²+x=6

And if we move the 6 to the opposite side..
x²+x-6=0

Why what do we have here? A parabola! Not too terrifying is it?

Okay so lets place the "<" back.
x²+x-6<0

Before actually solving this equation, it would help to understand what infact the question is asking. Well what we're trying to do is-
"Find the values of X where this parabola will have negative values"

So first we must factor the equation we came up with
x²+x-6<0
(x+3)(x-2)<0



Once we get the two roots, you must draw the line graph (it will be included for marks for the final answer). Finally pick any point in each of the 3 regions and sub it into the equation.

Answer: (-3, 2)
Remember! we use round brackets "( )" because we know the -3 and 2 are not included because the original equation did not include "≤" (less then AND equals to)



Question 2:
2x-3=5

Remember! The values in the absolute signs "x" can be both negative and positive, leaving us with two cases.

Case #1
2x-3=-5
2x=-2
x=-1 check: it works!

Case #2
2x-3=5
2x=8
x=4 check: it works!

Okay that was simple enough, BUT- what if we had..
2x-2>5

Yikes! Do not fear, remember- ignore the "<" and treat it as an "=" for now.

So we'd continue to solve just like before-
Case #1
2x-3>5
2x>8
x>4

Case #2
2x-3>-5
2x>-2
x>-1

Answer: (-∞,1)U(4,∞)
"U" - Union, that group together with this group

To be finished soon, very soon.
Sorry folks!

Homework assignment: All the questions you didn't get done on the past exercises (up to Exercise 28).
Mondays scribe will be Mel!isa

.
.
Enjoy your weekend!

Solving a System of Linear Inequalities

Sorry for the late post. Well, here's my scribe.

Solve the Following Linear Equation Graphically

Solve the Following Linear Inequalities
Hint: Answer is always graphically


Answer is the region highlighted ({), since its where the two regions meet.

Notice: that the lines (y < -x +3 , and y > 2x - 3) are dotted. This is because in linear inequalities a point(s) can only be in 3 areas. These are: BELOW the line, ABOVE the line, OR ON the line. In this case "y < -x +3" is below, and "y > 2x -3" is above.


Notice: that the point where the two lines meet is an open circle. This is because the two regions can go infinitely close to that point but they will never intersect at that point.

WHAT IF Y IS ALSO EQUAL TO THE LINE?

This Concept can be applied to any other shape (Circles, parabola, radical, etc.). Lets see Parabolas.

in Parabolas the region is either INSIDE, OUTSIDE or ON the parabola.
Example:
y <>

ONE MORE THING TO REMEMBER

When solving any inequality equation, if you divide/multiply by a negative, don't forget to change the direction of the inequality sign (<,>).

Example. When Solving 2x - y < 3
-y < -2x + 3 Divide both sides by a NEGATIVE.
y > 2x - 3

using the point (2,2)
Using the correct equation.
y > 2x -3
2 > 4 - 3 TRUE

BUT IF WE USE THE WRONG EQUATION.
y < 2x -3
2 < 4 - 3 FALSE Since 2 is greater, not less than 1

FINALLY...THE NEXT SCRIBE WILL BE me...jk....Its eedce. Have fun!

Applications with Systems of Linear Equations

Hi it's me ben again. Today in class Mr.K created a new rule to get people to participate in class and that's to pair up and let one person speak for the other person. Okay back to the math. Today we started with some Systems Of Linear Equations With Three Variable questions for practice.

a+2b+c=0
3a-b-2c=11
2a+b-c=3

3a+3b=3
5a+3b=11
2a=8
a=4

3(4)+3b=3
12+3b=3
3b=-9
b=-3

4+2(-3)+c=0
4-6+c=0
-2+c=0
c=2

EXPLANATION:
L₁
L₂
L₃
L₄=L₁+L₃
L₅=2L₁+L₂
L₆=L₅-L₄
L₇ Solve L₆
L₈ Solve L₄ w/ a=4
L₉ Solve L₈
L₁₀ Solve L₁ w/ a=4, b=-3
L₁₁ Solve L₁₀

Lines intersect @(4, -3, 2)

After a little work on that question we had to apply our Linear Equation skills we just learned and use them in word problems.

1.When 3 numbers are added in pairs the sums of the pairs are 28, 39, 45. What are the three number?
2Mark sells wildlife photos at the county fair, his revenue, R dollars is determined by the price, p dollars, that he charges for each picture as shown in the equation:
R(p)=ap²+bp+c
At a price of $40, his revenue is $2900
At a price of $50, his revenue is $3000
At a price of $70, his revenue is only $2600
Find the values of a,b, and c

Okay for #1 we need to find out the equation. So it states that the numbers are add in pairs so we need the possible combinations for the 3 equations and they are shown below then now solve.
a+b=28
a+c=39
b+c=45

-b+c=17
2c=62
c=31

b+31=45
b=14

a+31=39
a=8

EXPLANATION:
L₁
L₂
L₃
L₄=L₂-L₁
L₅+L₄
L₆ Solve L₅
L₇ Solve L₃ w/ c=31
L₈ Solve L₂ w/ c+31

Numbers are 8, 14, 31

For #2 we need to get another equation for each price and revenue. Notice that the price is p so just sub the price of each set of pictures and same with the revenues and you should get this:
a(40)²+40b+c=2900
a(50)²+50b+c=3000
a(70)²+70b+c=2600

Now just simplify and Solve
1600a+40b+c=2900
2500a+50b+c=3000
4900a+70b+c=2600

2400a+20b=-400
900a+10b=100
600a=-600
a=-1

900(-1)+10b=100
-900+10b=100
10b=1000
b=100

-1600+4000+c=2900
4000+c=4500
c=500

EXPLANATION:
L₁
L₂
L₃
L₄=L₃-₂
L₅=L₂-L₁
L₆=L₄-2L₅
L₇ Solve L₆
L₈ Solve L₅ w/ a=-1
L₉ Solve L₁ w/ a=-1, b=100

Therefore a=-1, b=100, and c=500

That's what we did today on Applications with Systems of Linear Equations and homework for tonight is EXERCISE #27 *But don't do #'s 1-5*
The next scribe will be M@RK and good night.

Systems of Linear Equations in Three Variables

Salutations,
Today in class, (for all you naughty kids that skipped, haha JK) we worked on equations with three variables. We did work on equations with two variables too. Well, here are the questions that we worked on:

3x+5y= 17
4x-y= -8
23x= -23
x= -1

4(-1)-y= -8
(-4)-y= -8
y= 4

EXPLAINATION:
L1
L2
L3= L1+5L2
L4 solve L3

L5 solve L2 w/ x= -1
L6 solve L6
Therefore lines intersect at (-1, 4)


4x+3y= 1
3x+2y= 2

-x= -4
x=4

3(4)+2y= 2
12+ 2y= 2
2y= -10
y= -5

EXPLAINATION:
L1
L2
L3= 2L1-3L2
L4 Solve L3
L5 solve L2 w/ x=4
L6 solve L5
Therefore, lines intersect at (4, -5)


-x+y+2z= -3
2x-y+ z= -7
x+2y-z= 7

3x+y= 0
-5x+3y=11
14x= -11
x= -11/14

3(-11/14)+y= 0
y= 33/14

2(-11/14)-(33/14)+z= 98/14
-22/14-33/14+z= 98/14
z= 153/14

EXPLAINATION:
L1
L2
L3= L1+5L2
L4 solve L3
L5 solve L2 w/ x= -11/14
L6 solve L5
L7 found x
L8 solve L4 for y w/ x= -11/14
L9 solve L8
L10 solve L2 for z with x=-11/14 and y= 33/14
L11 solve L10
L12 found z
Therefore lines interesect at (-11/14, 33/14, 153/14)



2x+y-3z= 9
x+y-2z= 7
zx-y+z= -7

3x-z= 0
4x-2z= 2
2x= -2
x= -1

3(-1)- z= 0
-3= z

x(-1)+y-2(3)= 7
-1+y+6= 7
y= -2


EXPLAINATION:
L1
L2
L3
L4= L2+L3
L5= L1+L3
L6= 2L4-L5
L7 solve L6
L8 solve L4 / x= -1
L9 solve L2 w/ x= -1 and z= -3
Therefore, the solution is (-1, 2, -3)


3x-2y+4z= 11
2x+3y-z= 5
x+4y-3z= 3

(1, 2, 3)
Are these solutions to that equation?

To solve this equation, all you have to do is sub in the numbers 1 for x, 2 for y, and 3 for z! Then, ta-dah there you have it.

3(1)-2(2)+4(3)= 11
2(1)+3(2)-3= 5
1+4(2)-3(3)= 3

As you can see, both L1 and L2 work out. However, the third line does not. Zero does not equal three, well last time I checked.

And, Ta-dah. That's what happened in math class today. Our homework for tonight is the numbers that were omitted from yesterday and number 26. Once again, our homework for tonight is numbers 1 and 2 from 25 and all of 26! Before I leave, I hold in my hand, the name of who will be the next scribe! The next scribe is.................................BENOFSCHOOL! Well, see you tomorrow everyone!

-SAMUS

Systems of Linear Equations in Two Variables

Ok, today in class we did something like the Friday's class. We practiced doing the different techniques on how to solve systems of linear equations in two variables.

We were given three systems to solve:

x=y+6
3x-5y=7

3x+2y=16
y+7x=19

2x+3y=4
5x+2y=3

Ok, lets do the first one. This first question is best suited to be answered using the substitution technique, because as you can see there is a equivalent for the x value.

x=y+6
3x-5y=7
3(y+6)-5y=7
3y+18y-5y=7
-2y=-11
y=11/2
x=11/2+6
x=11/2+12/2
x=23/2

Therefore, these lines intersect at (23/2,11/2).

Now, lets do the second one.This certain question is best suited to be answered using the elimination technique , because there is no equivalent on any of the variables unlike the first one.

3x+2y=16
y+7x=19

3x+2y=16
7x+y=19
-11x=-22
x=2
7(2)+y=19
y=5

Therefore,these lines intersect at (2,5).

Lastly, before we do some word questions. This question is suited to be answered using the elimination technique just like the second one.

2x+3y=4
5x+2y=3
-11x=-1
x=1/11
3y=4-2x
3y=44/11-2/11
3y=42/11
y=14/11

Here we go again with this word questions....

125 tickets were sold at a basketball game. A total of $337.50 was collected. Students paid $2.50/ticket and other people paid $3.50. How many students attended the game?

Let s=# of student's tickets
p=#of other people's tickets

2.50s+3.50p=337.50
s + p =125
-s =-100
s=100

Therefore , there are 100 student tickets and 25 other people's tickets.

After doing all the warm up things Mr. K started talking about consistent and inconsistent lines.
A consistent line means that there is an answer.It can be break up into a dependant and an independant line. Independant means that there is only one solution. Dependant means that there is an infinite amount of solutions. An inconsistent line means that there is no solution.

I'll be editing this tomorrow because the html codes for line 1 and line 2 doesn't seem to work.

The scribe for tomorrow is ............... "SAMUS" .

Sorry SAMUS ,but you're the one that pop into my hEAD first. I was gonna pick Lauressa but she said her computer wasn't working...

Homework for tonight is Exercise 25 excluding questions number 1 and 2.

Solving a System of Linear Equations

HEY! I'll just cut through the intro and get this scribe started.

On Friday's class we sort of started something new, but we still used some previous knowledge in order to work with the following questions.

We were given two equations.

x + y = 6
x - y = 2

y = -x + 6
x - 2 = y

Question: Do the lines intersect?
_________ Where?

It's better to know more than one way to solve a problem. You have to take on a certain equation in as many different angles as you can.

Mr. K taught us different ways to solve the equation.

There were 4 different techniques that he showed the class.

1. Graph : to actually draw out the equation on a graph
   
2. Comparison
   
3. Substitution
   
4. Elimination

*Important note: The Lattice Point is a point in the cartesian plane where the points are integers. (where the lines cross)

GRAPH

We've graphed the lines and can easily see where the lines cross (4,2). Graphing it will not always solve the problem because the point where the lines intersect won't always be a Lattice point.

COMPARING

At the Intersection, Y1 & Y2 are the same.

eg.)

y = -x + 6
x - 2 = y

-x + 6 = x - 2
____8 = 2x
____4 = x

This technique is called comparison. It's when 2 things equal to the same thing.


SUBSTITUTION


To figure out the rest, substitute 4 for x.

x + 4 = 6
4 + y = 6
y = 2

Lines intersect at (4,2)

ELIMINATION



OK...hopefully people will be able to understand this..

The Homework is Exercise 24

Next Scribe is: M@rk

didn't u read my scribe post yesterday at 11:18pm?

"the next scribe after this lesson was sandy. oh and dont pick me for scribe the next day cuz i was already picked for scribe. its just that my name hasnt yet been crossed off cuz my scribe wasnt yet posted but now it is. so after today's scribe, i think cheriee's next cuz her name hasnt yet been crossed off so she's the next and last scribe. good luck and you'll do a great job like always!!"

Analytic Geometry

Hello everyone!.
Today in class Mr. K said we were going to learn something new and other stuff we already knew about.

We worked on two questions:

1) If the distance from the line 2x-3y+8=0 to the line 2x-3y+K=0 is 24/√13 units find the value(s) of K.

2) Given:A (-1,3)
B (0,5)
C (-2,6)

a) Is ABC a right triangle?
b) Is ABC isosceles?
c) If M is the midpoint of AB and N is the midpoint of AC prove that MN // BC
d) Find a point D so that ABCD is a parallelogram
e) is ABCD a rectangle?

We were given some time to work on it. Most of the class had trouble with the first question like where to start.

* For the first question we talked about ways to find out if the lines were parallel ( // ).We knew that if the slopes were the same they are //.

some examples shown.

a) 2x-3y+8=0
4x-6y-k=0
* multiplied by 2

b) 2x-3y+8=0
-8x-12y+k=0
* multiplied by -4

-back to solving question #1

1) We changed the first line to be written in Standard Form

2x-3y+8=0 | 3y=2x+8
y=2/3x+8/3

From changing it to standard form we found the y intercept and the x intercept.

y intercept (0, 8/3)
x intercept (-4,0)

We looked at the question again and wrote down all the information we knew.

P (-4,0)
A= 2
B=-3
C=K
D=24/√13

Now that we know all of this information we just replaced them in the formula to find a distance from a point to a line.
D = | Ax + By + C | / √A2 + B2

24/√13 = | 29(-4) -3 (0) +K | / √4+9

24/√13 = | -8+k | / √13

24 = | -8+K |

24= -8+K -24 = -8+K

32= K -16 = K

The answer to this question has 2 Values, which are 32 and -16.


The second question wasn't all answered, but this is what i got.




2)

D = √(x2 -x1)2 + (y2-y1)2

AB=√1+4
=√5

BC =√4+1
=√5

CA =√9+1
=√10

(√5)2 + (√5)2 =√10

a) ABC is a right triangle
b) ABC is not a isosceles


* If anyone has more answers to this question feel free to comment and I'll update it. =)

Today we also wrote in our dictionaries, most of the information we already know.

My Analytic Geometry Tool Kit

The Pythagorean theorem | a2 + b2=c2

Slope of a line (a.k.a. rate of change) | (tri)y/(tri)x = y2 -y1/x1- x2

Midpoint of a line segment | midpoint = (x1-x2/2 , y1+y2/2)

Distance between two lines | D = √(x2 -x1)2 + (y2-y1)2

Distance between a point and a line | D = | Ax + By + C | / √A2 + B2

Equation of a lines

General Form | Ax+By+C=0

Standard Form | y=mx+b

Point-slope form | y2-y1=m(x2-x1)

Parallel lines (//) have equivalent slopes | m1-m2

Perpendicular lines have slopes which product is (-1) | m1-m2=-1

**Homework for today is exercise #23, and last the scribe for tomorrow is......-Zeph!!! Have fun!

Algebra Review

ALGEBRA REVIEW
LAST SCRIBE POST REVIEW BEFORE THE PRE-TEST!!!

in class, mr.k gave us equations to solve and two word problems.


SOLVING FOR X IN A GIVEN EQUATION


solve for x:

x + 3 = 4 - x

[(2x + 2)/(x^2 + 2x - 15)] + [2/(x - 3)] = 1/(x + 5)

√(w + 5) = √(3 - w) + 1


SOLVING FOR X IN AN ABSOLUTE EQUATION

x + 3 = 4 - x

since we are solving for x in an absolute value equation, x can only equal a positive number because the absolute value represents the distance and distance is always postive. because an absolute value of one side equals the absolute value on the other side, then there is more than one answer meaning that there are 4 equations.

we write down all the possible scenarios and since the absolute value operation means that whatever the number is inside the absolute value brackets, we're only look for its positive. for example, the absolute value of -3 is 3; OR -3 = 3.

mathematics is the science of patterns...

mr. k showed us that theres a pattern when solving for x in an absolute value equation. these are the possible solutions:

first, we remove one expression's absolute value brackets and there we are left with either a positive or a negative of that expression.

here are the possibilitites:

x + 3 = 4 - x;

x + 3 = -(4 - x);

-(x + 3) = 4 - x;

-(x + 3) = -(4 - x);

since we're looking for the positive value, all of these expressions have to be greater than 0.

wow...thats a lot of work just to solve for x but mr. k has lots of neat tricks up his sleeves so he showed us a shortcut.


"x + 3 = -(4 - x)" is the same things as "-(x + 3) = 4 - x" because all whats different is that a -1 was multipled on one side.

"x + 3 = 4 - x" is the same thing as "-(x + 3) = -(4 - x)" because all whats different is that a -1 was multipled on one side.

so really, we only need to solve for x for 2 equations which are:

x + 3 = 4 - x AND x + 3 = -(4 - x)


for x + 3 = 4 - x...

x + 3 = 4 - x
2x + 3 = 4
2x = 1
x = 0.5


for x + 3 = -(4 - x)...

x + 3 = -4 + x
x + 3 = x - 4

notice anything abnormal?

if we subtract both sides by x, then we get 3 = -4 which doesnt make sense.
if we add 4 to both sides we get 7 = 0 or if we subtrace 3 on both sides we get -7 = 0.

THIS IS AN EXTRANEOUS SOLUTION!

so for that equation, x = { }, N/A (not applicable), or "No Solution"

so x can only equal 1/2. BUT! we have to check if 1/2 fits perfectly into the given equation and it makes sense. so we sub in 1/2 for x.

x + 3 = 4 - x
(0.5) + 3 = 4 - (0.5)
3.5 = 3.5

both sides of the equation are equal so x = 3.5


in solving for x in an absolute equation, if the equation was like: x + 3 = 4 - x + 1, we have 4 different equations cuz a -1 isnt being multiplied on both sides. solve it and you'll see what i mean.

its like that because we are not looking for the absolute value of 4 - x + 1. we are solving for x when an absolute value equals another absolute value plus a number.

the next scribe after this lesson was sandy. oh and dont pick me for scribe the next day cuz i was already picked for scribe. its just that my name hasnt yet been crossed off cuz my scribe wasnt yet posted but now it is. so after today's scribe, i think cheriee's next cuz her name hasnt yet been crossed off so she's the next and last scribe. good luck and you'll do a great job like always!!