why do we get extraneous solutions? (INTRO)

Hey everyone.
In the last few days we've been looking at radical equations, and when solving these equations (roots) we sometimes get extraneous roots. Well, this class was about: Why do we get these roots in rational equation.

LETS LOOK AT THESE Qs

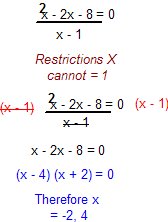
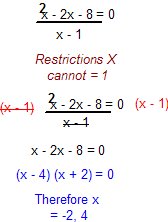
This means: the graph can't touch 1 and has roots when x=-2 and 4
i.e.


Graphically this is what is going on

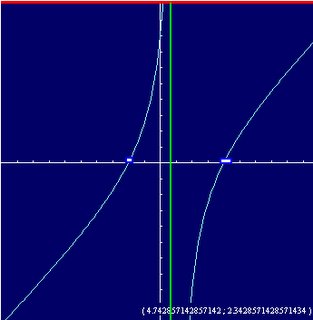The green line is called the ASYMPTOTE. Its a line that goes as close as possible to the restricted value (in this case 1) but never touches it. Therefore you can have an output when x=0.999 or x=1.111 but not when x=1

HOW ABOUT IF WE WANT

In our case here, lets first see the sign changes in the intervals between -infinite to -2; -2 to 1; 1 to 4; and 4 to infinite, in order for us to determine when the equation < style="text-align: center;">
1st interval -ve infinite to -2
pick any number in the interval e.g -12
plug into the formula

2nd interval -2 to 1
pick any number in the interval e.g 0
plug it into the formula


3rd interval 1 to 4
pick any number in the interval e.g 2
plug it into the formula

4th interval 4 to infinite
e.g 1000 000
plug it into the formula
OBVIOUSLY the answer is positive